Задача 45229 Решить неравенство ...
Условие
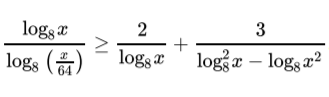
Решение
{x>0
{[m]log_{8}x ≠ 0 ⇒ x ≠ 1[/m]
{[m]log_{8}\frac{x}{64} ≠ 0 ⇒ \frac{x}{64} ≠ 1⇒ x ≠ 64[/m]
[m]log^2_{8}x-log_{8}x^2 =log^2_{8}x-2log_{8}x[/m]
Замена переменной:
[m]log_{8}x=t[/m]
[m]\frac{t}{t-2}\geq \frac{2}{t}+\frac{3}{t^2-2t}[/m]
[m]\frac{t}{t-2}- \frac{2}{t}-\frac{3}{t(t-2)}\geq0[/m]
[m]\frac{t^2-2(t-2)-3}{t(t-2)}\geq 0[/m]
[m]\frac{t^2-2t+1}{t(t-2)}\geq 0[/m]
[m]\frac{(t-1)^2}{t(t-2)}\geq 0[/m]
Решаем методом интервалов:
___+___ (0) _–__ [1] __–__ (2) ___+___
t < 0 или t=1 или t>2
Обратно:
[m]log_{8}x<0[/m] или [m]log_{8}x=1[/m] или [m]log_{8}x> 2[/m]
[mx < 1[/m] или [m]x=8[/m] или [m]log_{8}x>log_{8}64[/m] ⇒ x>64
C учетом ОДЗ:
О т в е т. (0; 1) U{8}U(64;+ ∞ )
