Задача 38018 Найдите длину l дуги кривой линии...

flaer

7 июня 2019 г. в 11:02

Найдите длину l дуги кривой линии √x+√y = √a, заключенной между точками касания ее с осями координат

sova

9 июня 2019 г. в 12:30

Запишем кривую в параметрическом виде:
x(t)=acos⁴t
y(t)=asin⁴t

тогда
√x=√acos²t
√y=√asin²t

Складываем
√x+√y=√a·(cos²t+sin²t)

√x+√y=√a

Поэтому применяем формулу:
L= ∫ t2t1sqrt ( ((x`(t))<sup>2</sup>+(y`(t))2 )dt

x`(t)= 4acos<sup>3</sup>t·(cost)`=4acos³t·(–sint)
y`(t)=4asin<sup>3</sup>t·(sint)`=4asin³t·cost

(x`(t))<sup>2</sup>+(y`(t))²=16a²·(cos⁶t·sin²t+sin⁶t·cos²t)=

=16a²·sin²tcos²t·(cos⁴t+sin⁴t)=

формула понижения степени: sin²x=(1–cos2x)/2;cos²x=(1+cos2x)/2⇒

sin⁴t+cos⁴t=(sin²t)²+(cos²t)²=(2+2cos²x)/4=(1+cos²x)/2


=4a²·(sin2t)²·(1+cos²2t)/2=2a²(sin²2t)·(1+cos²2t)


При x=0
t₁=π/2

При х=а ⇒ acos⁴t=a ⇒ cos⁴t=1 ⇒ cos²t=1;
cost=1
t₂=0

∫ ⁰_π/2 = – ∫ ^π/2₀


L= – ∫ ^π/2₀ √2a·sin2t·√1+cos²2tdt=

=√2a ∫ ^π/2₀√1+cos²2td(cos2t)/2=

=(√2/2)· a· (cos2t+√1+cos²2t+ln|cos2t+√1+cos²2t)/2|) | ^π/2₀=


=a·(√2/4)(0 + +ln|cosπ+√1+cos²π)/2|–ln|cos0+√1+cos²0)/2|=

=a·√2ln(√2–1)/8– a√2·ln(√2+1)/8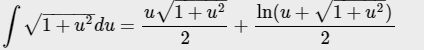

vk201218220

7 июня 2019 г. в 14:44

x=0 y=a
y=0 x=a
l= ∫√1+y'²dx от 0 до a
y'=1– √a/x
l= ∫√2–2·√a/x+a/xdx