Задача 49829 [r][blue][b]Пожалуйста помогите решить...
Условие
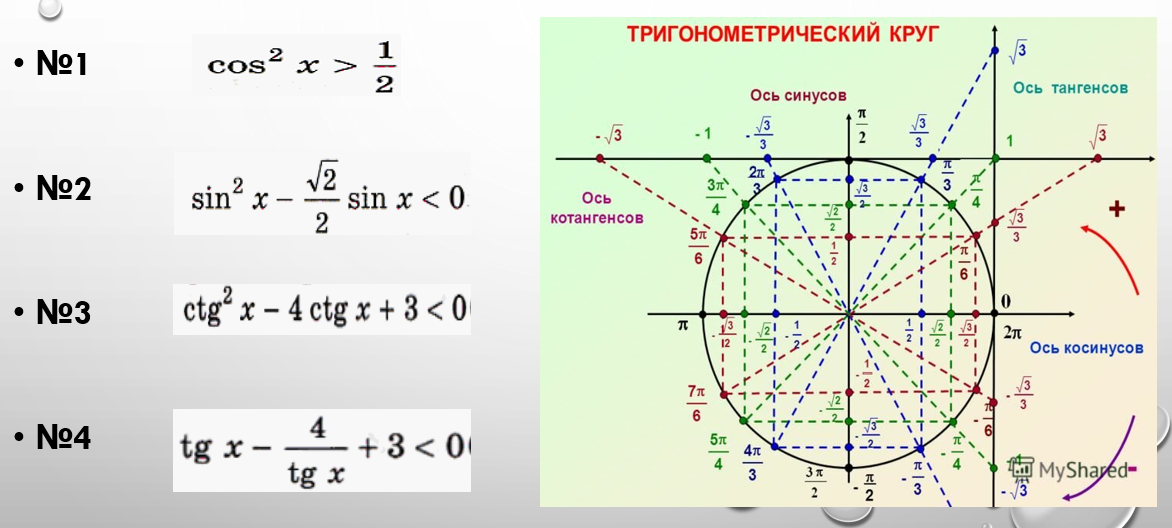
Решение
[m]\begin{matrix} cosx<- \frac{\sqrt{2}}{2}& ;cosx >\frac{\sqrt{2}}{2} & \end{matrix}[/m]
[m]\begin{matrix} \frac{3\pi}{4}+2\pi n <x<\frac{5\pi}{4}+2\pi n & ;-\frac{\pi}{4}+2\pi n <x<\frac{\pi}{4}+2\pi n, n \in Z & \end{matrix}[/m]
2.
[m]\begin{matrix}sinx\cdot (sinx- \frac{\sqrt{2}}{2}) <0& \end{matrix}[/m]
[m]\begin{matrix}sinx <0 &; sinx> \frac{\sqrt{2}}{2}& & \end{matrix}[/m]
[m]\begin{matrix}\pi +2 \pi n < x < 2 \pi +2\pi n, &; \frac{\pi}{4}+2\pi n <x< \frac{3 \pi}{4}+2\pi n, n \in Z& \end{matrix}[/m]
3.
ctgx=t
t^2-4t+3 <0
D=16-4*3=16-12=4
t_(1)=1; t_(2)=3
1 < t < 3
1 < ctgx < 3
y=ctgx - убывающая функция, бОльшему значению функции соответствует мЕньшее значение аргумента
[m]\begin{matrix}arctg3 + \pi n < x < \frac{\pi}{4}+\pi n , n \in Z& \end{matrix}[/m]