Задача 30650 Решите систему...
Условие
system{x+y=π/4; tgxtgy=5–2√6}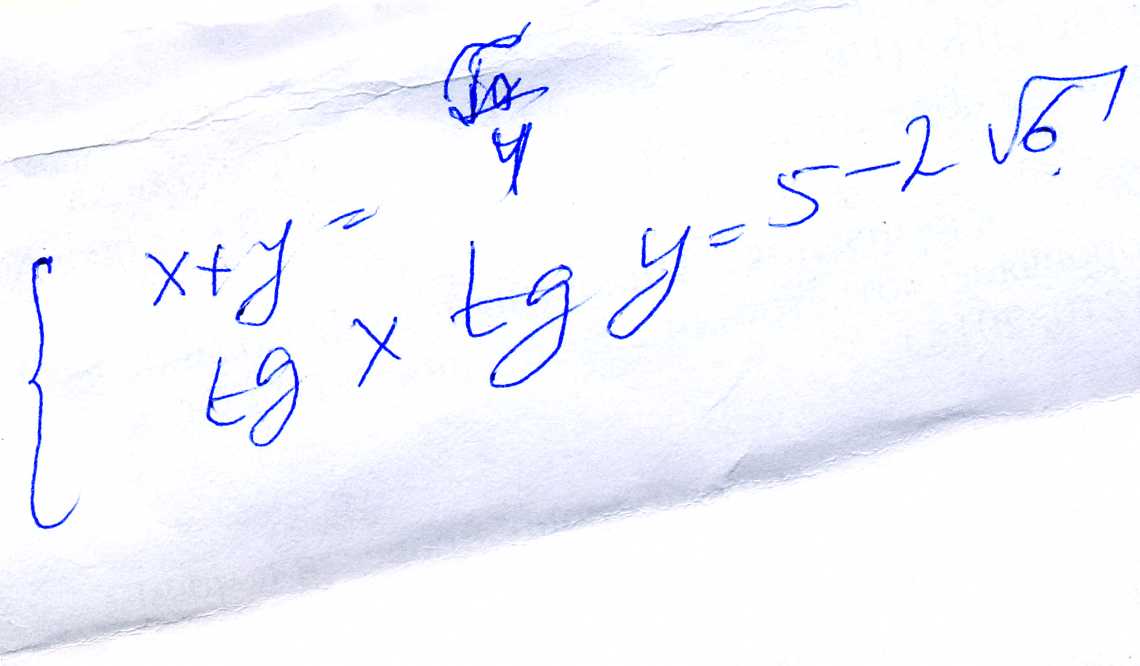
математика 10-11 класс
1314
Решение
★
{tgx·tg((π/4)–x)=5–2√6
tg((π/4)–x)=(tg(π/4)–tgx)/(1+tgx·tg(π/4))=(1–tgx)/(1+tgx)
tgx·(1–tgx)/(1+tgx)=5–2√6;
tgx·(1–tgx)=(5–2√6)·(1+tgx);
tgx–tg2x =5–2√6+5tgx –2√6·tgx
tg2x+(4–2√6)tgx +(5–2√6)=0
D=(4–2√6)2–4·(5–2√6)=16–16√6+24–20+8√6=
=20 –8√6=4·(5–2√6)
tgx=2–√6–√5–2√6 или tgx = 2–√6+√5–2√6
x1 =arctg(2–√6–√5–2√6)+πn, n ∈ Z или x2=artctg( 2–√6+√5–2√6)+πm, m ∈ Z
y1=( π/4)–arctg(2–√6–√5–2√6)–πn, n ∈ Z или y2=( π/4)–arctg(2–√6+√5–2√6)–πm, m∈ Z
Обсуждения